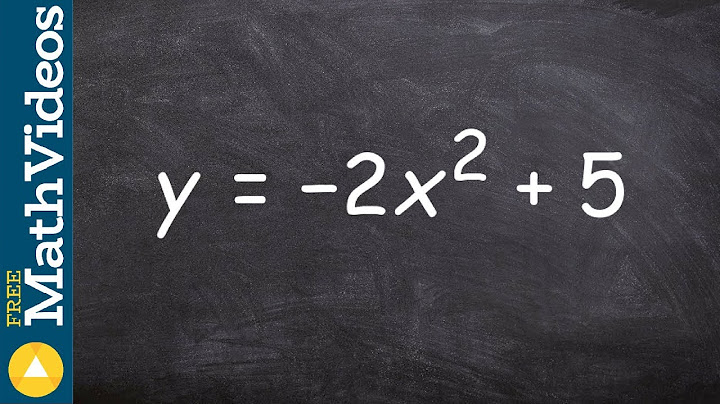Equation of parabola with focus and directrix

As we saw in Quadratic Functions , a parabola is the graph of a quadratic function. As part of our study of conics, we'll give it a new definition. A parabola is the set of all points equidistant from a line and a fixed point not on the line. The line is called the directrix, and the point is called the focus. The point on the parabola halfway between the focus and the directrix is the vertex. The line containing the focus and the vertex is the axis. A parabola is symmetric with respect to its axis. Below is a drawing of a parabola.

If a parabola has a vertical axis, the standard form of the equation of the parabola is this: (x - h)2 = 4p(y - k), where p≠ 0. The vertex of this parabola is at (h, k). The focus is at (h, k + p). The directrix is the line y = k - p. The axis is the line x = h. If p > 0, the parabola opens upward, and if p < 0, the parabola opens downward.

If a parabola has a horizontal axis, the standard form of the equation of the parabola is this: (y - k)2 = 4p(x - h), where p≠ 0. The vertex of this parabola is at (h, k). The focus is at (h + p, k). The directrix is the line x = h - p. The axis is the line y = k. If p > 0, the parabola opens to the right, and if p < 0, the parabola opens to the left. Note that this graph is not a function.

The Greeks defined the parabola using the notion of a locus. A locus is a set of points satisfying a given condition. These points will generally lie on some curve. For example, the circle with centre \(O\) and radius \(r\) is the locus of a point \(P\) moving so that its distance from the point \(O\) is always equal to \(r\).

The locus definition of the parabola is only slightly more complicated. It is very important and gives us a new way of viewing the parabola. One of the many applications of this is the refection principle, which we will look at in a later section.

We fix a point in the plane, which we will call the focus, and we fix a line (not through the focus), which we will call the directrix. It is easiest to take the directrix parallel to the \(x\)-axis and choose the origin so that it is equidistant from the focus and directrix. Thus, we will take the focus at \(S(0,a)\) and the directrix with equation \(y=-a\), where \(a > 0\). This is as shown in the following diagram.

We now look at the locus of a point moving so that its distance from the focus is equal to its perpendicular distance to the directrix. Let \(P(x,y)\) be a point on the locus. Our aim is to find the equation governing the coordinates \(x\) and \(y\) of \(P\).

The distance \(PS\) is equal to \(\sqrt{x^2 + (y-a)^2}\), and we can see from the diagram that the perpendicular distance \(PT\) of \(P\) to the directrix is simply \(y+a\).

Since \(PS=PT\), we can square each length and equate, giving

The last equation may also be written as

and so we see that it is the equation of a parabola with vertex at the origin.

The positive number \(a\) is called the focal length of the parabola.

Any parabola of the form \(y=Ax^2+Bx+C\) can be put into the standard form

with \(a > 0\), where \((p,q)\) is the vertex and \(a\) is the focal length. When a parabola is in this standard form, we can easily read off its vertex, focus and directrix.

Example

Find the vertex, focal length, focus and directrix for the parabola \(y=x^2-4x+3\).

Solution

We complete the square and rearrange as follows:

Hence the vertex is \((2,-1)\) and the focal length is \(\dfrac{1}{4}\).

The focus is then \((2, -\dfrac{3}{4})\) and the equation of the directrix is \(y=-\dfrac{5}{4}\).

A parabola may also have its directrix parallel to the \(y\)-axis and then the standard form is \((y-p)^2 = \pm 4a(x-q)\), with \(a > 0\).

Exercise 13

Put the parabola \(y^2-2y= 4x-5\) into the above standard form (for a directrix parallel to the \(y\)-axis) and thus find its vertex, focus and directrix, and sketch its graph.

What is the focus and Directrix formula?

What is parabola using focus and Directrix?

How do you find the equation of the Directrix of a parabola?